报告题目:On linear preservers between matrices over an arbitrary field
报告人:黄毅青 教授 台湾中山大学

邀请人:刘 磊
报告时间:2023年11月10日(周五)下午14:30-15:30
报告地点:腾讯会议598-998-219
报告人简介:黄毅青 (Ngai-Ching Wong) ,台湾中山大学教授。黄毅青在泛函分析、算子代数领域开展了卓有成效的研究工作,获得了丰硕的研究成果。2004年,黄毅青教收受华人数学家大会 ICCM 2004邀请做45分钟报告。黄毅青教授发表SCI论文一百多篇,同时在多份著名SCI数学期刊,如Operators and Matrices, Linear and Multilinear Algebra, Banach Journal of Mathematical Analysis等, 担任编辑委员。
报告摘要:Let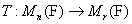 be a linear map between matrices (maybe of different sizes) over an arbitrary field
be a linear map between matrices (maybe of different sizes) over an arbitrary field 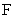 . Many preserver problems can be formulated as the one preserving matrices annihilated by a fixed polynomial
. Many preserver problems can be formulated as the one preserving matrices annihilated by a fixed polynomial , i.e.,
, i.e.,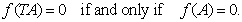 For example, if
For example, if , the above is equivalent to that
, the above is equivalent to that 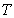 preserves idempotent matrices; while if
preserves idempotent matrices; while if 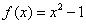 , the above is equivalent to that
, the above is equivalent to that 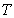 preserves involution matrices. We find that when the domain
preserves involution matrices. We find that when the domain 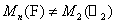 , the zero set of
, the zero set of 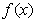 is ``simple enough'' and
is ``simple enough'' and 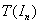 is central in the range of
is central in the range of 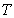 , any such linear preserver
, any such linear preserver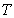 assumes a canonical form, namely,
assumes a canonical form, namely, 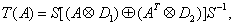 for some invertible
for some invertible  , and diagonal matrices
, and diagonal matrices 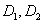 of appropriate sizes. The diagonal entries
of appropriate sizes. The diagonal entries 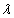 of
of 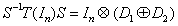 satisfy the multiplier condition
satisfy the multiplier condition 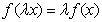 . Here, by saying
. Here, by saying 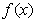 is ``simple enough'' we mean that the zeroes of
is ``simple enough'' we mean that the zeroes of 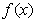 does not form an additive coset of
does not form an additive coset of 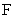 . The result is indeed a consequence of our new finding that any disjoint idempotent preserver
. The result is indeed a consequence of our new finding that any disjoint idempotent preserver 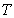 assumes such a canonical form when the domain of
assumes such a canonical form when the domain of 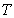 is not
is not 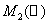 .
.